Form of the particular solution

First derivative of partic. soln

Substitute in the diff eqn

==>

and

Equate coefficients of similar terms

==>

Using Mathcad to solve for A and B

So, we can solve for A and B. But what about C?.

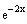
The coefficient C can not be determined because the term appears in both the nonhomogenus term and the complementary solution.

Example

Solve the diff eqn

The terms and derivatives of the nonhomog functions are

, , , , , ,

Non appear in the complementary solution. Therefore the form of the particular solution is

CASE 2 (The terms of f(x) or its derivatives appear in the complementary solution)

Example (failing)

Solve

Solution:

Associated homog. eqn

Roots of the charac. poly

Complementary solution

1 , x and

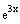
Nonhomgenous terms and their derivatives

So the general solution is given by

Check

Example

If the roots of the characteristic poly of the diff eqn

are 4, 4, 4 , 2i, -2i. Find the form of the complementary solution of the diff eqn

Solution

Particular solution before modification

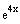
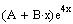
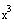
Since appears in the complementary solution with multiplicity 3, so we multiply by . Similarly the cos 2x and sin 2x appear in the comp solution with multiplicity 1. so we multiply the terms of the sin and cos by x.

Thus, the modified particular solution is

Solution

Assoc homog eqn

Roots of the char poly

complementary solution

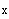
If we ignore the fact that the term appears in the complementary solution, then the particular solution will have the form

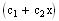
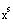
To avoid duplicating the terms that appear in the complementary solution we multiply the corresponding terms in yp(x) by , where s is the multiplicity of the root that produced the duplicated

terms (in this case 2) (See the justification page 330 in text)

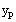
Thus, we modify by multiplying it by

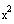
Substitute the derivatives in the diff eqn gives

==>

The above eqn says that every other solution of the nonhomogenous eqn is the sum of the associated solution of homogenous eqn and a particular solution of the nonhomogenous eqn.

Thus, to find the general solution of the nonhomogenous eqn we first solve the associated homogenous eqn and try to find one particular solution of the nonhomogenous eqn.

Method of Undetermined Coefficients

This method will work of nonhomogenous linear diff. eqns with constant coefficients with the nonhomogenous term f(x) that are linear combinations of products of the functions

1. polynomials in x

2. erx

3. cos(bx) and sin(bx)

Examples of f(x)

The method of solution depends on wither the terms of the function f(x) or any of their derivatives appear in the solution of the associated homogenous eqn.

CASE 1 (non of the terms of f(x) or their derivative appear in the solution of the homog. eqn)

Example

Solve the diff eqn

y'' + 4y = 3x - 2 e-x

Solution:

y'' + 4y = 0

The associated homog. eqn.

5.5 Undetermined Coefficients and Variation of Parameters

The general nonhomog linear diff. eqn has the form

(1)

with the associated homogenous eqn

(2)

Let

(3)

Then eqn (1) can by written as

The operator L defined in (3) is a linear operator. That is

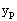
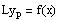
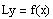
Let be a particular solution of the nonhomogenous eqn (1), i.e., . If y is any other solution of eqn (1), i.e., then

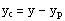
This means that the difference of the two solutions is a solution of the associated homogenous eqn. (2)

==>

==>

and

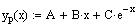
Therefore, when substituting in the form of the particular solution , we get

And the general solution is given by

Example

Find the form of the particular soln of the diff eqn.

Solution

Associated homog. eqn

Roots of the charac. poly.

Complementary solution

Roots of the characteristic eqn.

The complementary solution

The terms and their derivatives of the nonhomogenous function f(x) =3x - 2 e-x are

1, x and e-x

Note that non of them appear in the complementary solution. Therefore, let us try a particular solution of the form

where A and B are coefficient to be determined by substituting in the diff Eqn.

==>

Substitute in the diff. eqn.

Equating the coefficients of similar terms gives