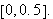Taylor Polynomials with Remainder
In all the following exercises,

![]() is taken as
is taken as

![]()
Let

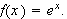 The 5th Tayor polynomila for
The 5th Tayor polynomila for

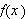 is
is
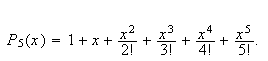
and
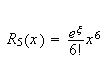
Using
these formulas, or otherwise, find find

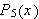 and for
and for

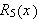 for the functions
for the functions

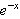

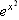
Use

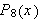 for the function
for the function

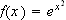 to approximate
to approximate

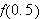 and
and

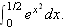 Determine the accuracy of this approximation knowing that
Determine the accuracy of this approximation knowing that

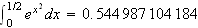 .
.
Determine

![]() for
for

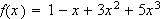 and for
and for

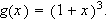
For

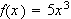 find
find

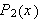 and
and

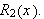 Use your results to approximate
Use your results to approximate

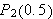 and give the error exacly and using the formula for
and give the error exacly and using the formula for

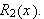
Find

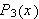 for
for

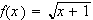 and use your results to approximate
and use your results to approximate

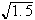 and
and

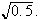 Which result is a better approximation of the corresponding true value?
Suggest a way to obtain better accuracy for the less accurate result.
Which result is a better approximation of the corresponding true value?
Suggest a way to obtain better accuracy for the less accurate result.
Find

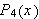 for
for

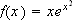 and then use your results to estimate the accuracy acheived when using
and then use your results to estimate the accuracy acheived when using

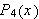 to approximate
to approximate

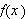 on the interval
on the interval

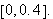
In the previous exercise, aproximate

![]() and
and

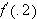 by replacing
by replacing

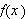 by
by

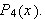
For the function

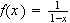 find
find

 and
and

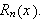 Find the Taylor polynomial of least degree to approximate
Find the Taylor polynomial of least degree to approximate

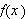 to
to

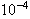 accuracy on the interval
accuracy on the interval

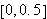 and use it to approximate
and use it to approximate

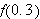
On an Excel sheet draw the graphs of the functions

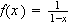 and its Taylor polynomials
and its Taylor polynomials

![]() over the interval
over the interval